Math for Motorcycles #3 — Power and Torque

This is an article in my series on math for motorcyclists.

This article is about power and torque and how they’re related to engine rpm, top speed, and thrust.

If you’ve ever read a spec sheet for a motorcycle, car, or truck, then you’ve seen power and torque figures. But I’m sure you’ve wondered the same things I’ve wondered, like:

• How does a high power figure relate to what it feels like to ride a certain bike or drive a certain car?
• Do two vehicles with the same peak power accelerate at the same rate?
• Why do some vehicles have more torque than power, like low-revving cruisers for example?
• What’s the difference between a kW, a horsepower, and a bhp… are they all the same thing? Maybe just the last two?

And many other things.

To start with, let’s look at what power and torque are. This is making me think back to my high school physics. Luckily, I’ve done all that for you so you don’t need to dust off those expensive textbooks you never managed to throw away…

Note: I’ve continually made updates to this, and corrected some parts of it, thanks to people writing in. Some updates include corrections to the definition of force, and a clearer explanation of what happens when you change sprockets.

What is torque?

Let’s start with the easy one: torque.

Torque is “twisting force”. If you apply a unit of force to a lever, then you are applying torque.

Some online commenters say “torque is acceleration”. That’s a murky sentence that would upset many physics teachers, but without getting into semantics: Separate out the words torque (twisting ability) and thrust (pushing forward ability). Torque does impact thrust, but so does gearing.

Engine torque, after gearing and so on, becomes thrust, which is what you feel wrenching you forward. It’s a more visceral word for “acceleration”.

Some real life examples of torque:

• You spin a merry-go-round by turning it at the outside, not at the centre somehow. You only need to apply a little bit of force a few times and soon it is spinning quite fast.
• You tighten a nut by applying pressure to the end of a wrench, not the bit closest to the nut. This gives you more twisting power, or torque.

Torque is measured in force multiplied by distance, which provides leverage. This is reflected in the units used to describe torque:

• Metric: One Newton-metre (Nm) of torque is a Newton of force (which is the force required to accelerate a kilogram at 1 m/s²) applied with the leverage of one metre.
• Imperial: One foot-pound / pound foot (ft-lb or lbf-ft) of torque is a pound of force (the force of a pound of weight at sea level, a.k.a. lbf) applied with the leverage of one foot.

Note: There’s another, non-standard metric unit of force, called a kilogram-force, which is defined as the force on a kilogram in standard gravity. One unit of kilogram-force is around 9.8 Newtons. (Hey, don’t get mad at metric! There’s all kinds of weird stuff in imperial too… like British and US definition of gallons, and measuring horses in “hands”. We all get along via tire size definitions, which bring us together in a cozy mess of imperial and metric units, together at last…

Nomenclature — in metric units, the unit for force is a newton and the unit of weight is a kilogram. In imperial units, they both have the same name — pound. So you have to clarify whether you mean a pound of weight (“lb”), or a pound of force (“lbf”). Similar, but different.

Because a pound of force is bigger than a Newton of force (1 lbf = 4.45 N) and a foot is smaller than a metre (one foot = 0.305 m), a foot-pound is similar in magnitude to a Newton-metre — 100 foot-pounds = 134 Newton-metres. (See more on converting between metric and imperial units).

Torque in a motorcycle (or car or truck) engine is the ability of the engine to twist.

In motorcycle specs from manufacturers, the torque is measured at the crankshaft.

For example, a motorcycle with 100 Nm of peak torque can twist something 1 metre away with 100 Newtons of force. If you move it to two metres away, it’ll only be able to push it with 50 newtons of force.

Similarly, a motorcycle with 100 lbf-ft of force can twist something at the end of a one-foot pole with 100 lb of force. If it’s a 10 foot pole, it’ll only be able to apply 10 lbf of force.

Why measure the length of the pole when thinking of the impact of torque?

The crankshaft has a few things to get through before it gets to the tyre. Each of these change the impact of the torque, through gearing.

Torque / distance (including multipliers) = force. The torque starts at the engine, goes through gears, sprockets/the shaft, and the wheel + tyre, and becomes force against the surface of the road (or dirt).

The things the engine goes through to get to the surface:

1. Gears. The gears have a ratio that will multiply the torque by some factor. The higher the gear, the lower the force.
2. Sprockets or shaft. In a motorcycle, there’s sprockets (for chain and belt drives) or a shaft with a built in ratio.
3. Wheel/tyre. There’s a wheel at the other end with a tyre on it.

All of those things — gears, sprockets/shaft, or wheel/tyre, change how the torque feels on a bike. You might read that gearing changes affect the “thrust” of a bike.

That’s why on a Suzuki SV650, which has relatively little torque compared to a big cruiser like a Suzuki M109R for example, you can still accelerate away from the lights pretty quickly. Both have similar zero-to-freeway acceleration times (around 4 seconds depending on rider skill, traction, shifting), despite being hugely different bikes.

See here for a longer discussion on torque, gearing, and thrust — why little bikes can still accelerate quickly.

So for example, let’s assume the sprockets of a motorcycle are in the ratio of 15:45, with 15 on the front sprocket, and 45 teeth on the rear. This is actually quite similar to most middleweight and above motorcycles.

This means the front-to-rear ratio is 1:3. So the front sprocket has to turn three times for the rear sprocket to turn once.

The effect of this is to slow down the rear wheel. But it also acts as a lever on the torque on the front wheel, multiplying it by three. So the front sprocket might be turning at 240 rpm with 500 Nm (already geared up via the primary drive and gearbox), but the rear sprocket will then be turning at 80 rpm with 1500 Nm.

If you remove a tooth from the front sprocket and add one to the rear — a common modification that roughly preserves the same chain length — you change the drive ratio.

The ratio becomes 14:46, which is 1:3.286, a roughly 10% increase in the drive ratio. Now, the front sprocket has to turn 3.286 times to turn the rear sprocket. All other things being equal, for the same rpm, the rear wheel will be turning around 10% slower, but will have around 10% more torque — and thus force, and acceleration.

If you did the opposite — add teeth in the front sprocket, or remove teeth from the rear — it has the opposite effect, of reducing torque, but increasing top speed.

Should you add teeth to the front sprocket or remove them from the rear? There are lots of considerations — things like chain clearance, sprocket clearance, and, having to change the length of the chain. It’s case-dependant — no clear-cut answer. (And there are no good studies on the effect of wear of doing either, just a lot of anecdotal personal experience.)

See my general guide to motorcycle gearing for more tools to help think about it.

Similarly, if you add a bigger rear wheel or thicker profile tyre, it’ll reduce acceleration and increase top speed.

What is power?

This is easier. Power is money, and time is money, so power is… time.

Jokes aside, power is defined as energy transfer per unit of time. Power in metric units is defined as the “watt”, which is defined as one “joule” of energy per second.

Power is kind of bandied around in the vehicle world, and generally used to mean a “feeling”. But two bikes with similar power specs can respond extremely differently to a twist of the throttle.

That’s why it’s possible to do wheelies and burnouts on a Honda Grom, which has a paltry 7 kW (9 hp) of power, and do the same things on motorcycles with 10-20 times that figure.

Basically, you can’t just take a power spec and know what it means for how a motorcycle behaves. Yes, more power definitely usually correlates to more acceleration and more fasterness, but there’s more to it!

To start with, think about the definition: Power is defined as energy transfer per time. But exactly what are you transferring energy into?

In a kettle, you transfer power into heat (of the water). In a light, you transfer power into light (and heat). But what about in a vehicle — where does the energy go?

The energy of a moving motorcycle is kinetic energy. The basic way a motorcycle works is it takes potential energy (from the fuel or battery) and converts it to kinetic energy. That kinetic energy is stored in the forward movement of the motorcycle and the rotational movements of the wheels and other moving parts.

The formula for kinetic energy is: K.E. = ½ m v²

This has some interesting consequences.

Firstly, the heavier the object, the more energy you need to get it moving the same speed. So if your bike + rider is 1000 lb (say on a fully loaded Gold Wing), you need twice the energy to get it to highway speed, than say on a 500 lb bike + rider (say on a race bike with a small rider).

Secondly, the energy in a moving vehicle is proportional to the square of the speed.

This means

• An object going twice the speed has four times the kinetic energy. So if it takes X energy to get something to 30 mph, it takes another 3X the energy to get to 60 mph.
• A bike (or car or whatever) going 100 mph has roughly twice the energy in it of one going 70 mph.

That’s the main reason that acceleration slows. You need to pour in increasingly more energy to get something to go incrementally faster. This is true even in a world with no resistance or friction.

The second reason acceleration slows — outside a perfect physical system — is because of resistance.

In a perfect physical system (a vacuum with no friction), once you put energy into an object and it starts moving, it’ll keep moving forever. For example, once a probe is sent off into space to explore other planets, it doesn’t need more energy to keep boing, because nothing is slowing it down.

That’s why in movies (and in real life, though I haven’t had the pleasure) when a space craft is in a vacuum in space, it shuts off the engines and keeps going. There’s no friction to slow it down.

So why do you need to keep burning petrol (or using battery charge if you’re on an electric) just to keep going forward at the same speed? The answer is to overcome friction forces.

If you let go of the throttle, the following friction forces will slow you down:

• Air (which you may know as air resistance or drag)
• Tyres (a.k.a. “tires”) against the ground
• Friction in the moving parts of the motorcycle — axels, drivetrain, engine

So like how with torque you can change the impact of torque by changing drive ratios and gearing, with power you can change the impact of power by using

• A more aerodynamic body (fairings) — this is the biggest factor
• Low-friction tyres (which are bad for cornering or acceleration, but good for top speed)
• Lower friction engine components (hard to do)

Above low suburban speeds, the friction of air (drag/air resistance) is by far the biggest source of friction. So to get more leverage out of engine power, making your machine more aerodynamic is your biggest leverage factor. That’s why vehicles that beat land speed records look like this:

Drag increases exponentially as you get faster. According to the drag equation:

• F_D = ½ ρ v² C_d A

Drag force increases in proportion to the square of velocity.

Since the power equation says that P = F•v (force times velocity), the power needed to overcome drag is proportional to the cube of velocity.

• P_D = F_D•v = ½ ρ v³ C_d A

So in a nutshell, just to overcome drag, you need a lot more power to go from 2-300 km/h than you need to go from 1-200 km/h. That’s why you can get to 60 mph/ 100 km/h on a Honda Grom with 7 kW / 9 hp of power, but you’ll need a lot more than double that (14 kW or 18 hp) to get to 120 mph/200 km/h.

Common units of power: What are bhp, hp, PS, CV, Ch, and kW?

See also my article on quick conversions between units of power.

When reading motorcycle specs, we often see many of these units.

There are three main units of power in modern vehicle stats.

Firstly, there’s bhp or brake horsepower, which is what we conventionally call imperial horsepower. This is an imperial unit used only in North America (mostly the US; Canada is technically metric, but culturally there’s some overlap).

Brake horsepower is a technical definition of 33000 lbf-ft/min. It translates to 178.1074 calories per second of energy.

1 bhp ≈ 745.700 Watts or about 0.75 of a kW. As a shortcut I say 100 bhp 75 kW.

Secondly, there’s “metric” horsepower.

You see metric horsepower quotes in mostly European spec sheets (from Ducati, BMW, etc.). The numbers look very similar, so many don’t know the difference. And in documentation from Europe in English, they’re often described as just “horsepower”.

Technically, metric horsepower is defined as the power to raise a mass of 75 kg against the Earth’s gravitational force over a distance of one metre in one second.

This ends up being about 735.5 W, slightly less than one bhp.

So 100 bhp / imperial horsepower = 101.4 metric horsepower. And 100 metric horsepower is about 98.6 bhp.

Metric horsepower is commonly quoted as:

• Just “hp” in European documents (e.g. by Japanese or European manufacturers, but publishing in Europe)
• CV in Italian or Spanish — short for cavalli vapore or caballos vapor, which means “horse steam”. The term dates back to the time of steam engines.
• PS in German — short for Pferdestärke, or “horse power”
• ch in French — short for chevaux, “horses”
• Л.С. in Russia — short for лошадиная сила, “horse power”

All those units of metric horsepower are the same.

Finally, there’s kW or kilowatts. You see this most often in the Asia/Pacific region (Japan, Australia, New Zealand, Singapore, China, etc.).

The Watt has a much simpler technical definition: one joule of energy per second.

Fundamentals: Power = Torque x RPM (x a constant)

Anyone who has seen a dyno chart will note that torque stays roughly flat, while RPM goes up.

See this dyno chart for example:

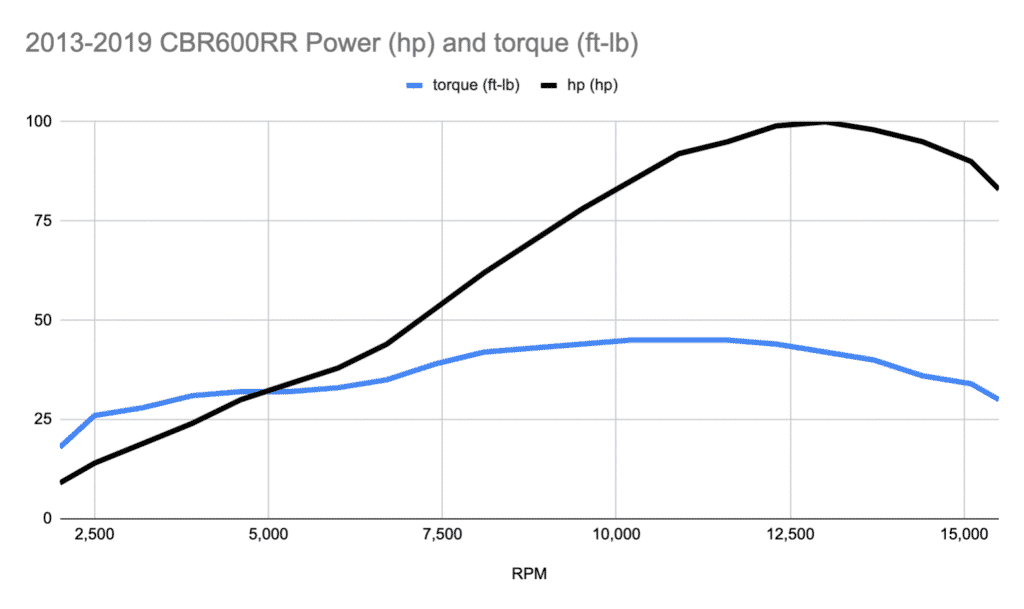
In the above chart, torque does increase, but it’s relatively flat compared to the power curve.

The reason for that is the basic power formula: P = F•v, Power = force x velocity. Applied to rotating objects (angular motion), this becomes P = T•v.

So you multiply torque by rotating speed to get to power. Of course they’re all in different units, so you need some constants for conversion between units.

• Metric: Power (kW) = Torque (Nm) x speed (rpm) / 9549
• Imperial: Power (hp) = Torque (ft-lb) x speed (rpm) / 5252

For example, if I say my bike makes 50 Nm at 3000 rpm, then you can know that this translates to 50 x 3000 / 9.5488 = 15.7 kW of power.

Or if I make the same claim in imperial units, and say it makes 37 ft-lb, then you can say 37 x 3000 / 5252 = 21 hp.

If you know your quick shortcut conversions between metric and imperial units (in this case that kW is about 3/4 of hp), then you’d know those both look about right.

This isn’t peak power by the way. It’s just how much power is being generated at that point. It’d be one point on a curve.

Torque, Horsepower, and What a Bike is Like to Ride

There’s a reason that Harley-Davidson rarely quotes power figures, and usually only quotes torque figures: because torque often tells you more of the story — if you make certain assumptions.

The reason torque tells you more of the story is that if you assume a few things, torque translates to “pulling power below high speeds”. Let me justify this.

Here are the assumptions to make:

1. Assume a fairly “normal” gearing ratio on the bike, no matter the drivetrain, including front and rear sprockets, gear ratios, and wheel sizes.
2. Assume a “normal” RPM range. For any given size or class of engine, this is also a safe assumption (e.g. big bore bikes rev less, small bore bikes rev higher, assuming same number of cylinders)
3. Maybe even assume all the bikes are V-twins (which they mostly are for cruisers, which Harley-Davidson riders are mostly shopping for)

So with those assumptions, for most road bikes, you have to operate in the first four gears until you’re on the highway, when you can relax in fifth or sixth gear.

If you assume those things, and then I tell you “This V-twin road bike makes a peak of 120 ft-lb, whereas this V-twin cruiser makes a peak of 80 ft-lb”, you know that the 120 ft-lb one is going to be more arm-wrenching when you crack the throttle.

The assumptions are a little looser when you compare different engine sizes or designs. If I say “This bike has 150 Nm peak torque” and “this bike has 100 Nm of peak torque”, and the first is a liquid-cooled inline-four and the second is a V-twin… it’s still comparable if they’re both big-bore road bikes.

This is the case because big-bore road bikes (not track-oriented sport bikes, not small capacity bikes) are tuned to produce a wide spread of torque across a range of RPMs. So if you assume that two very different designed engines are both aiming for a wide RPM range for road use, then you can compare peak torque figures with reasonable accuracy.

Let’s look at those torque curves to understand more. In some buyers guides, I compare torque of sport bikes (more track-oriented machines) and roadsters (more road-oriented bikes).

Have a look at this below chart comparing the torque curves of the 847cc CP3 engine (from the previous generation MT-09/Tracer 900/XSR900), and that of a late-model Yamaha YZF-R1:

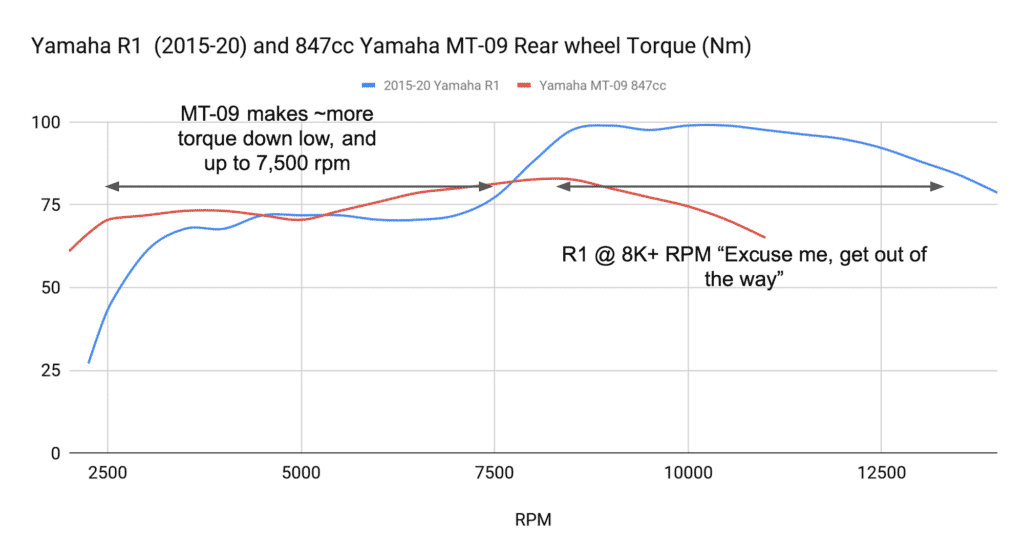
The annotations tell the story. But basically — the MT-09 is a road bike, and starts plateauing after 8K rpm. The R1, meanwhile, just gets started around there.

Where you can’t compare peak torque is comparing a road bike to a superbike. It just doesn’t make sense.

For example, The Harley-Davidson Iron 1200 makes peak torque of 100 Nm (73 ft-lb) at 3,500 rpm… but runs out of puff between 4-5K, when you should shift (if not before then). Many sport bikes (like Ducatis) are flat-out unpleasant under 3K rpm.

Comparing torque or even power of two very different classes of bikes doesn’t tell you anywhere near enough to know what they’re like to ride.

What horsepower tells you… and where it falls short

So why do we keep using horsepower?

The briefest of answers is that horsepower does tell you about the acceleration.

The more powerful an engine, the more power it can pour into the body in the form of kinetic energy.

Even though there’s a lot of nuance (how heavy the bike is, what the torque curve is like), a high-power bike does feel fast.

Secondly, horsepower tells you about top speed.

Again, this is making an assumption of two bikes having similar aerodynamics. But most motorcycles that aim for high speeds have roughly similar aerodynamics. To help these go faster, keeping everything else constant, you need more power.

The final reason we use horsepower is that it’s a shorthand for what a motorcycle can achieve. It’s a shorthand. It says “This bike probably produces a lot of torque at a humanly achievable RPM”.

So if you assume that the RPM range of two bikes is vaguely similar and something you’d use in everyday life, then the horsepower figure tells you roughly how arm-wrenching the torque will be, how wheelie-prone the bikes will be, and overall how “fast feeling” the two bikes are.

This is most true when comparing very different numbers. So if I compare a 150 kW (200 hp) superbike with a 35 kW (50 hp) commuter, it’s obvious from the figures alone that the former is much faster than the latter. It’s going to feel more bonkers, have much more pull, and a much higher top speed.

But if I compare two bikes with similar power figures and ignore the other differences, I’m likely to get into trouble.

For example, take the previous generation MT-09 (with the 847cc engine) and the BMW R nineT. Both these mikes make similar peak claimed power — roughly a 5% difference between them.

But there’s so many other things different between these two bikes:

• Style of engine (inline triple vs boxer twin)
• Number of cylinders (3 vs 2)
• Coolings system (liquid vs air/oil) — impacts how high the engine can rev
• Drivetrain (wet clutch + chain vs dry clutch + shaft)
• Gearing (in the gears and sprockets/shaft)
• Diameter of tire

All of these mean you can’t simply say one bike will be as fast as the other. They feel eminently different to ride for the above and other reasons.